==> %body 
==>
 
%body
%body
%body %body
%body %body %body
%body SINGULAR - A Computer Algebra System for Polynomial Computations Overview Objects Functionality Libraries Examples Applications Availability History Contributors Future %body
Sao Carlos, 08/02 http://www.singular.uni-kl.de
SINGULAR Examples Build. Blocks Comb. Appl. HCA Proving
Arrangements Branches Classify Coding Deformations Equidim Part Existence Finite Groups Flatness Genus Hilbert Series Membership Nonnormal Locus Normalization Primdec Puiseux Plane Curves Saturation Solving Space Curves Spectrum %body
Sao Carlos, 08/02 http://www.singular.uni-kl.de
SINGULAR Applications Robotics Circuit Design Medicine Glass Melting %body
Sao Carlos, 08/02 http://www.singular.uni-kl.de
A line in projective 3-space through the points
(x0:x1:x2:x3) and (y0:y1:y2:y3)

has Plücker coordinates
pij = xiyj - xjyi.


In this way, lines in P3 correspond to points in P5 satisfying the equation
p01p23-p02p13 +p03p12=0.

The equation for a general line p to meet a fixed line q is linear in these coordinates:
p01q23-p02q13 +p03q12 +p12q03 -p13q02 +p23q01=0.

The equations for a line to be tangent to a quadric is
.
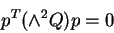
Back
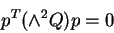