|
 |
 |
 |
 |
 |
 |
 |
 |
 |
5.1.164 vandermonde
Syntax:vandermonde (ideal_expression,ideal_expression,int_expression)Type:- poly
Purpose:- vandermonde(p,v,d) computes the (unique) polynomial of degree
d with prescribed values v[1],...,v[N] at the points
p
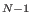p
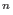, N=(d+1)
, 
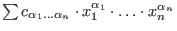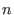the
number of ring variables.
The returned polynomial is
, where the coefficients
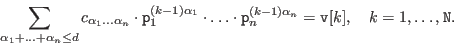are the solution of the (transposed)
Vandermonde system of linear equations
![\begin{displaymath}\sum_{\alpha_1+\ldots+\alpha_n\leq d} c_{\alpha_1\ldots\alpha...
...\tt p}_n^{(k-1)\alpha_n} =
{\tt v}[k], \quad k=1,\dots,{\tt N}.\end{displaymath}](sing_186.png)
Note:- the ground field has to be the field of rational
numbers. Moreover, ncols(p)==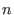, the number of variables in the
basering, and all the given generators have to be numbers different from
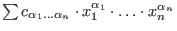0,1 or -1. Finally, ncols(v)==(d+1), and all given generators have
to be numbers.
Example:ring r=0,(x,y),dp; // determine f with deg(f)=2 and with given values v of f // at 9 points: (2,3)^0=(1,1),...,(2,3)^8=(2^8,3^8) // valuation point: (2,3) ideal p=2,3; ideal v=1,2,3,4,5,6,7,8,9; poly ip=vandermonde(p,v,2); ip[1..5]; // the 5 first terms of ip: ==> -1/9797760x2y2-595/85536x2y+55/396576xy2+935/384x2-1309/3240xy // compute value of ip at the point 2^8,3^8, result must be 9 subst(subst(ip,x,2^8),y,3^8); ==> 9