
Compute the (first and second) Hilbert series of a homogeneous ideal.
|
Twisted cubic in P3 :
1st Hilbert series: This result can be used to compute the Hilbert polynomial H of
M = K[x,y,z,w] / I :
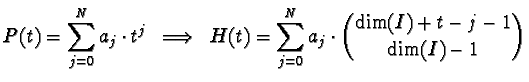
Hilbert polynomial: |