|
|
7.6.2 Groebner bases for two-sided ideals in free associative algebras
We call a total ordering 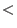 on the free monoid on the free monoid
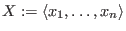 (where (where 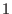 is identified with the identity element) a
monomial ordering if the following conditions hold: is identified with the identity element) a
monomial ordering if the following conditions hold:
-
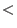 is a well-ordering on is a well-ordering on  , that is , that is 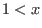
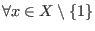 , , -
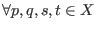 , if , if 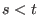 , then , then
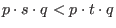 , , -
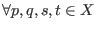 , if , if
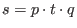 and and 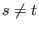 , then , then  . .
Hence the notions like a leading monomial and a leading coefficient transfer to this situation.
We say that a monomial
 divides monomial divides monomial
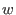 , if there exist monomials , if there exist monomials
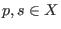 , such that , such that
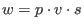 . .
In other words
 is a proper subword of is a proper subword of
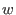 . .
For a subset
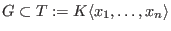 ,
define a leading ideal of ,
define a leading ideal of 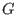 to be the two-sided ideal to be the two-sided ideal
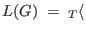
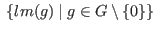
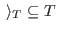 . .
Let 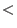 be a fixed monomial ordering on be a fixed monomial ordering on  .
We say that a subset .
We say that a subset 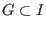 is a (two-sided) Groebner basis for the ideal is a (two-sided) Groebner basis for the ideal  with respect to with respect to 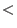 , if , if 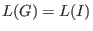 . That is . That is
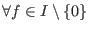 there exists there exists 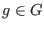 , such that , such that
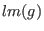 divides divides 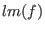 . .
|